Gravity in 2001: A Space Odyssey
April 23, 2012 in Editorial
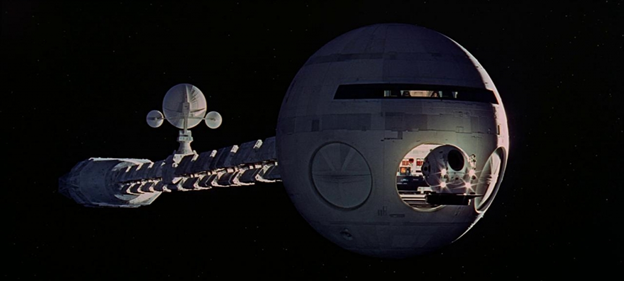
Aside from the classic problems of the Fridge Door Light and the Awkward Facebook Friend Request, there is another issue that keeps many of us awake at night. How do we exercise in Space? 2001: A Space Odyssey gave us an answer – simply have the astronauts run in a centrifuge that simulates gravity. About an hour into the movie we see one space jogger moving around quite comfortably. In fact, he appears to be moving with the same amount of ease as someone jogging on Earth, leading Centives to wonder: is the centrifuge spinning fast enough to generate a replacement for Earth’s gravity?
Using some creative math that we’ve outlined below, Centives figured out that the gravity that the astronauts felt in the centrifuge was just 4% of Earth’s gravity. This means that the gravity seen in the spacecraft is just a quarter of the gravity on our Earth’s very own moon – and the astronauts that visited the lunar surface had a fair amount of trouble getting around there. Our space protagonist shouldn’t be able to jog the way he does, unless those tight shorts conceal a considerable amount of added mass.
In fact, according to our estimates, in order to generate earth level gravity the centrifuge would have to spin slightly more than 12 times a minute, or five times as fast as it currently spins.
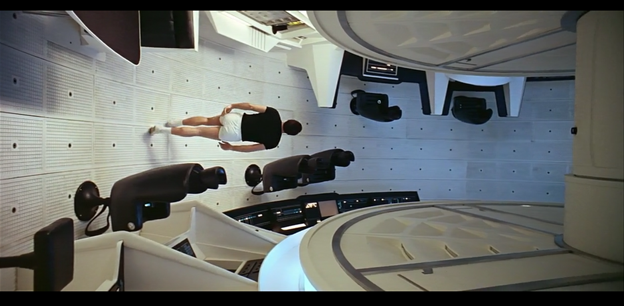
Another thing that the film makers seem to have glossed over is that since the man’s height is around one third the centrifuge’s radius, his feet are (relatively speaking), much further from the center than his head. Thus, his feet will be experiencing a much greater force, making it difficult for him to move normally.
If you’re more technically minded and want to know how we came to our conclusions, we started by figuring out the value of a few key variables.
First we took a look at the speed of the centrifuge. In the film, the character in question takes from 56:16 to 56:28 to run half the circumference of the centrifuge. We also know that he is, in fact, running in place as the wheel rotates around him. Thus, the angular velocity of the centrifuge can be estimated to be ∏ radians per 12 seconds.
To determine the radius of the centrifuge, we took the still frame above and measured the distance of the character and the radius of the centrifuge in pixels. The man is around 160 pixels and the centrifuge is 515 pixels. By estimating the man’s height to be 1.776m (average height of an American male), we estimated that the centrifuge had a radius of 5.73m.
We then plugged these values into the formulas outlined below. Click through the slideshow to see our steps. Hit the button on the lower left corner to expand it.
Enjoyed reading this? You might also like our look at the the physics of the Hulk saving Iron Man, or the value of TARDIS. You can find those and other interesting things in our Editorials although if you want a selection of our most popular check out the Greatest Hits. You might also want to follow us on Twitter or Facebook to get the latest and greatest from Centives.
So there were a number of problems in 2001 regarding gravity. In the beginning, it seems like the director went to great lengths to portray gravity, but when the astronauts get near the moon, everything was normal. The cruising ships seemed to have earth gravity, they ate snacks and sandwiches normally. The moonbase also was inhabited by people who operated in earth gravity. This was a disappointment. Also on the Jupiter mission, gravity was inconsistent. I understand the need not to make the hamster wheel 4 football fields tall. It was amazing enough to build what they built. Still, a little more concentration on the moon scenes would have been nice and not too difficult. I’m surprised it was glossed over quite frankly given the extreme attention to detail in this movie.
the formulas file is out
anyway thanks for making the numbers
good job keep on
science fan from madrid
Thanks so much for pointing that out Emilio. We’ve updated it so that it works.